How to measure distances at sea and on land? When you stand on a mountain or a tall building, you can see just as far as the Phoenician sailor once did, high in the crow's nest on the mast.
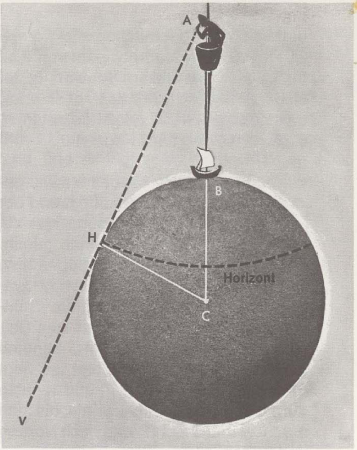
We draw the globe and on it, exaggeratedly large, a man in a crow's nest, as he looks around, as far as he can see. On the drawing the man is point A.. Its height above the earth is then AB. The line BC is the radius of the earth, a line from the center to the surface. It is round 6368 Kilometers long. The man looks along the AHV line to point H., Where the sky and the sea seem to meet. Only at this point does the line touch the earth. When he looks around, as far as he can see, these points of contact together form a circle on the globe. This circular line is called the horizon. (Such a large part of the world, as indicated by the horizon line drawn here, only sees a man in reality, when it is in a satellite several thousand km from Earth.) Does the seaman want to measure the distance to the horizon, so he uses the mathematical formula d = 2,081 times √h. Here d means the distance to the horizon in nautical miles and h the height above the sea surface in meters. Let's try an example:
The man is in the masthead of a ship 9 Meters above the sea surface. How far is the horizon? We use the formula 2,081 · √9. The square root of 9 is 3 (3 • 3 = 9). So our answer is 2,081 • 3 = 6,243 Nautical miles or, there one nautical mile = 1,852 Kilometer is, 6,243 • 1,852 = 11,562 Kilometer.